Fig.1: (a-b) Different Hall configurations.ρzy, the out-of-plane Hall configuration with the field along x axis.ρxy, the in-plane Hall configuration with the field alongzaxis. (c) Comparison of out-of-plane (ρzy) and inplane (ρxy) Hall responses. ρzy consists of two parts, the anomalous Hall resistivity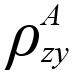 
with a spontaneous value of 4.2 μΩ cm at 0 T, and the ordinary Hall resistivity 
with a value of 0.77 μΩ cm when the field is swept from 14 T to 0 T. ρxy, looking like ordinary Hall effect attains 3.9 μΩ cm at 14 T, exceeding by far. (d) Field dependence of ρxy with temperature varying from 100 to 400 K. (e) Temperature dependence of ρxy and at 14 T. (f) Temperature dependence of field-induced linear anomalous Hall resistivity 
. (g) Temperature dependence of field-induced linear anomalous Hall conductivity 
. It is monotonously increasing with cooling.
Background
Understanding the origin of anomalous Hall effect (AHE), observed as early as 1881 in ferromagnetic solids1 has been enriched in the present century by considering the role played by the topology of electron wave-function. In a uniform magnet, the Berry curvature leads to anomalous velocity as a fictitious magnetic field in the momentum-space, which exhibits monopole-like texture around the Weyl point, and is extensively regarded the intrinsic source of AHE. In unconventional magnetic structures like skyrmions, an electron hopping on non-coplanar spin lattice with spin chirality picks up a real-space Berry phase and also lead to a Hall response, which is commonly referred to as the topological Hall effect (THE). Both AHE and THE are characterized by a non-linear Hall resistivity. To the best of our knowledge, there is no report on anomalous Hall response without significant departure from field-linearity, believed to be a necessary ingredient for separating ordinary and unusual components of the Hall response.
What we discover?
In this work, by measuring the transverse electric and thermoelectric coefficients up to 14 T, we show that the in-plane field-linear Hall number of Mn3Sn, is five times larger than what is expected from the carrier density and the Nernst signal is two orders of magnitude larger than what expected given the mobility and the Fermi energy of the system. We reveal an additional hidden component dominating the ordinary signals in both cases. Our theoretical calculations reveal that out-of-plane spin canting44 induced by the magnetic field leads to nonzero spin chirality and simultaneously generates Berry curvature by gapping Weyl nodal lines unrecognized before. Our results present a unified mechanism between the real-space and momentum-space Berry phases as the origin of this unusual field-linear Hall effect.
Why is this important?
NC editor's summary:Berry curvature sits at the heart of both the anomalous Hall effect and topological Hall effect, with the former arising from a momentum space berry curvature, while the latter arises from a real space Berry curvature. Here, Li et al present an intriguing example of a combined real and reciprocal space Berry curvature in the kagome material Mn3Sn, resulting in a large field linear anomalous Hall effect.
Who did the research?
Xiaokang Li1,*, Jahyun Koo2, Zengwei Zhu1,*Kamran Behnia3, Binghai Yan2,*
(1)Wuhan National High Magnetic Field Center and School of Physics, Huazhong University of Science and Technology, Wuhan 430074, China
(2)Department of Condensed Matter Physics, Weizmann Institute of Science, Rehovot, 7610001, Israel
(3) Laboratoire de Physique et d’Etude de Matériaux (CNRS-Sorbonne Université)
ESPCI, PSL Research University, 75005 Paris, France
Link
https://www.nature.com/articles/s41467-023-37076-w
Funding
This work was supported by the National Science Foundation of China (Grant No.51861135104 and No.11574097) and The National Key Research and Development Program of China (Grant No.2016YFA0401704). B.Y. acknowledges funding from the European Research Council (ERC) under the European Union’s Horizon 2020 research and innovation programme (ERC Consolidator Grant ``NonlinearTopo'', No. 815869). K. B was supported by the Agence Nationale de la Recherche (ANR-18-CE92-0020-01; ANR-19-CE30-0014-04). B.Y. and K.B. acknowledge a research grant from the Potter's Wheel Foundation / Weizmann-CNRS collaboration program. X. L. acknowledges the China National Postdoctoral Program for Innovative Talents (Grant No.BX20200143) and the China Postdoctoral Science Foundation (Grant No.2020M682386).
